In this lesson I will show you how to factorise x^36x^211x6=0 through simple observationCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyIf no integer roots are found, try out fractions x=1 x = 1 By Factor theorem, xk is a factor of the polynomial for each root k Divide x^ {3}6x^ {2}11x6 by x1 to get x^ {2}5x6 Solve the equation where the result equals to 0 By Factor theorem, x − k

Which Is The Graph Of The Function F X X 3 6x 2 11x 6 Brainly Com
F(x)=x^3-6x^2+11x-6 g(x)=x-3
F(x)=x^3-6x^2+11x-6 g(x)=x-3-By inspection, x = 1 is a zero so (x1) is a factor By synthetic division x^3 6x^2 11x 6 = 0 → (x 1)(x^2 5x 6) = 0 For the quadratic factor 6 = 2*3 → 23 = 5 The quadratic factors nicelyF(1) = 4(1) 3 6(1) 2 8(1) 6 = 4 6 8 6 = 0 Getting it




Two Zeroes Of The Polynomial X3 6x2 11x 6 Are 2 And 3 Find The Third Zero Maths Polynomials Meritnation Com
How do you factor x^3 6x^2 11x 6 = 0 ?If the function f(x) = ax^3 bx^2 11x 6 satisfies the conditions of Rolle's theorem for the interval 1,3 and f'(2 1/√3) = 0, then the values of a and b are respectively In each of the following use factor theorem to find whether polynomial g x is a factor of polynomial f x or not f x x 3 6x 2 11x 6 g x x 2 3x 2 Tutorix
Factorise x 3 6x 2 11x 6 completely using factor theorem remainder; x^3 6x^211x 6 I want to factorize it what are easiest and quicktest way to find the factors ?G(x) = x – 3 Solution If g(x) is a factor of f(x), then the remainder will be zero that is g(x) = 0 g(x) = x 3 = 0 or x = 3 Remainder = f(3) Now, f(3) = (3) 3 – 6(3) 2 11 x 3 – 6 = 27 – 54 33 – 6 = 60 – 60 = 0 Therefore, g(x) is a factor of f(x) Question 2 f(x) = 3X 4 17x 3
Subtract 6 6 from 1 1 Multiply 11 11 by 1 1 Add − 5 5 and 11 11 Subtract 6 6 from 6 6 Since 1 1 is a known root, divide the polynomial by x − 1 x 1 to find the quotient polynomial This polynomial can then be used to find the remaining roots Divide x 3 − 6 x 2 11 x − 6 x 3 6 x 2 11 x 6 by x − 1 x If two of the zeroes of the polynomial f(x)=x44x3x2104x105 are 3√2 and 3√2,then use the division algorithm to find the other zeroes of f(x) 2x⁴x³14x²15x8 divide by x²3x2 Using division algorithm find the quotient and remainder of the following I) x³6x²11x6You can find polynomial roots for x^36x^211x6 on a calculator by just entering the polynomial expression in the input field and tap on the calculate button to get the result in no time ie, 1, 3, 2



Search Q The Factorization Of 6x2 2b 11x 2b 3 Is Tbm Isch



Q Tbn And9gcsjcugx5pkoowy3 Cjavlyoito0xc2svvhtwt2b8zeniasxlz9o Usqp Cau
Calculadoras gratuitas passo a passo para álgebra, trigonometria e cálculo611x6x^2x^3=0 2x^5x^42x1=0 116xx^2=\frac {6} {x} x^32x=0 2x^5x^42x1=0 polynomialequationcalculator 611x6x^2x^3=0 en And the answer should be x^3 6x^2 11x 6 and where we do not have to rearrange for that becomes the valid polynomial x^3 6x^2 11x = 6 and the function F(x) = x^3 6x^2 11x x F(x) 0 0 025 239 05 4125 075 5296 1 6 125




X 3 6x 2 11x 6 क ग णनखण ड क ज ए




Solve The Equation X 3 6x 11x 6 0 If The Roots Being In A P
Question 1 f(x) = x 3 – 6x 2 11x – 6;Click here👆to get an answer to your question ️ Factorise x^3 6x^2 11x 6Answer to Determine the highest real root of f(x)= x^3 6x^2 11x 61 by using the modified secant method Continue for three iterations and




Plzz Help Two Zeroes Of The Polynomial X3 6x2 11x 6 Are 2 And 3 Find The Third Zero Maths Polynomials Meritnation Com




Determine The Root Of F X X 3 6x 2 11x 6 1 Chegg Com
Let f(x) = x^3 6x^2 11x 6 Try x = 1: f(1) = 1 6 11 6 = 0 So x 1 is a factot of f(x) Try x = 2: f(2) = 8 24 22 6 = 0 If x2 and x1/2 both are the factor of the polynomials nx^2 5x m , then show that m=n Factorise The given Polynomial Find what must be subtracted from 4y412y36y250y26 so that obtained polynomial is exactly divisible by y24y2 Factorise 2u33u217u30 Using factor theorem factorise the polynomial x rays to 4 x rays to 3 7x rays Factorise x3 6x2 11x 6 using FACTOR THEOREM 2 See answers Advertisement Advertisement khanujarashmit khanujarashmit Solution is attached below Advertisement Advertisement Therefore, 1, 2, 3 are the factors of f(x) Advertisement Advertisement New questions in Math



How Do I Use Long Division To Simplify 12x 3 11x 2 9x 18 4x 3 Socratic




Solved Determine The Highest Real Root Of F X X 3 6x 2 11x 6 1 1 Answer Transtutors
X^{3}6x^{2}11x6=0 he Related Symbolab blog posts Practice Makes Perfect Learning math takes practice, lots of practice Just like running, it takes practice and dedication If you want (y is also equals to f(x))f(x)=x^36x^211x6In kennedymyrick kennedymyrick Mathematics College answered Use the xintercept method to find all real solutions of the equation x^36x^211x6 1 See answer kennedymyrick is waiting for your help Add your answer and earn pointsIf the Product of Zeros of the Polynomial F(X) Ax3 − 6x2 11x − 6 is 4, Then a = Mathematics Advertisement Remove all ads Advertisement Remove all ads Advertisement Remove all ads MCQ If the product of zeros of the polynomial f(x) ax 3 − 6x 2 11x − 6 is 4, then a = Options \\frac{3}{2




Q 16 Classify The Following Functions F X Defined In Rrarrr As Injective Surjective Both O Youtube



What Must Be Added To F X 6x5 5x4 11x3 3x2 X 5 So That It May Be Exactly Divisible By G X 3x2 2x 4 Mathematics Topperlearning Com 6ocvt9cnn
Determine all possible Jordan forms of the 2\times 2 matrices satisfying in p(x)=x^36x^211x6 Determine all possible Jordan forms of the 223 Find roots (zeroes) of F(x) = x 3 6x 2 11x6 Polynomial Roots Calculator is a set of methods aimed at finding values of x for which F(x)=0 Rational Roots Test is one of the above mentioned tools It would only find Rational Roots that is numbers x which can be expressed as the quotient of two integers TheYou can find polynomial roots for x^36x^211x6 on a calculator by just entering the polynomial expression in the input field and tap on the calculate button to get the result in no time ie, 1, 2, 3




If 2x 3 Is A Factor Of 6x 2 X A Find The Value Of A With This Value Of A Factorise The Given Expression



What Should Be Added In The Polynomial X 3 6x 2 11x 8 So That It Is Compleely Divisible By X 2 3x 2 Sarthaks Econnect Largest Online Education Community
Factorise x 3 6x 2 11x 6 completely using factor theorem Advertisement Remove all ads Solution Show Solution `"Let" f(x)=x^36x^211x6 ` `for=x=1 ` `f(1)=(1)^36(1)^211(1)6` `==1212=0 ` Hence, (x1) is a factory of f(x) ∴ `x^36x^211x6=(x1)(x^25x6) `Solution Here, f ( x) is a polynomial with integer coefficient and the coefficient of the highest degree term is 1 Therefore, integer roots of f ( x) are limited to the integer factors of 6, which are ± 1, ± 2, ± 3, ± 6 Therefore, x = 1 is not a zero of f ( x) Hence, the integral roots of f ( x) are − 1, − 2, − 354 Find roots (zeroes) of F(x) = x 4 6x 3 11x 2 6 Polynomial Roots Calculator is a set of methods aimed at finding values of x for which F(x)=0 Rational Roots Test is one of the above mentioned tools



Find The Integral Roots Of The Polynomial F X X 3 6x 2 11x 6 Sarthaks Econnect Largest Online Education Community




Let F R Rarr R Be A Function Such That F X X 3 6x 2 11x 6 Then F X Is
Then we can use synthetic division or long division to give us a quadratic factor to go with the (x n) Factoring a quadratic should be straightforward for you if you are working on factoring cubics For example f(x) = 4x 3 6x 2 8x 6 I try guessing x = 1 Does f(1) = 0?2 How do you find Polynomial Roots x^36x^211x6 on a calculator?Share It On Facebook Twitter Email 2 Answers 2 votes answered by AbhishekAnand (870k points) selected by Vikash Kumar Best answer Hence, (x 1) is a factor of f(x)




Factorising A Quadratic Like 6x 11x 3 Youtube
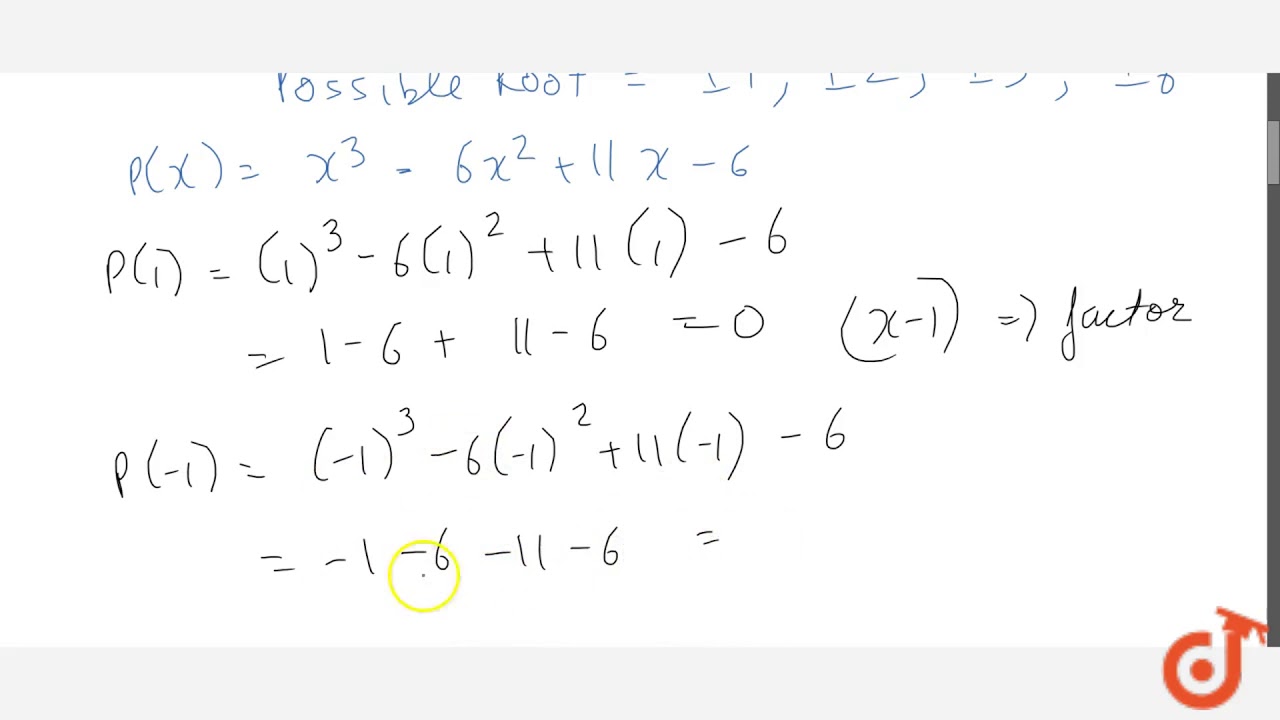



Using Factor Theorem Factorize The Polynomial X 3 6x 2 11x 6 Youtube
Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!I think that this is the xintercept method First of all, we set y=x^36x^211x6 To find x, we need to let y=0 (y is also equals to f (x)) f (x) = x^36x^211x6Find stepbystep Engineering solutions and your answer to the following textbook question Determine the highest real root of $$ f(x) = x^3 6x^2 11x 61 $$ (a) Graphically (b) Using the NewtonRaphson method (three iterations, $$ x_i = 35 $$ ) (c) Using the secant method (three iterations, $$ x_{i1}= 25 $$ and $$ x_i = 35 $$ )



Q Tbn And9gctacrs7vhr O Gxdcnblhyzzxno51hntzrawh5maqly8 6ci1nj Usqp Cau




Which Is The Graph Of The Function F X X 3 6x 2 11x 6 Brainly Com
Zero, because x^3–6x^211x6=(x1)(x2)(x3) is positive only when , and has at least three distinct factors then 325 views Submission accepted by Piyush BansalSimple and best practice solution for f(x)=x^36x^211x6 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itDetermine the root of f (x) = x^3 6x^2 11x 61 (a) Using the NewtonRaphson method (three iterations, x_i = 35) (b) Using the secant method (three iterations, x_i 1 = 25 and x_i = 35) (c) Using the modified secant method (three iterations, x_i = 35, delta = 001)



How To Factor This Equation Using Decomposition Quora




F X X 3 6x 2 11x 6g X X 2 Brainly In
The sum of the values of a for which $$\frac{x^36x^211x6}{x^3x^210x8} \frac a{30} = 0$$ does not have a real solution is A $1$ B $12$ C $13$ D $2$ I tried to factorise the numerator and Use the Remainder Theorem to completely factor p(x) = x^3 6x^2 11x 6 Please show your work 1 See answer Advertisement Advertisement shanepierce is waiting for your help Add your answer and earn points LammettHash LammettHash By the rational root theorem, we have several possible candidates for rootsDetermine the highest real root of f (x) = x^3 6x^2 11x 61 using the following techniques Graphically don't forget to always add a title and axis labels Add comment to your code identifying the solution, or add a text box to the figure Create a NewtonRaphson function and use it to solve this problem, with a starting value of x = 35



If A Is Domain Of F X In Tan 1 X 3 6x 2 11x 6 Sarthaks Econnect Largest Online Education Community




X3 6x2 11x 6 Divided By X2 X 1 Brainly In
Question Given f(x)=x^36x^211x6 Show that f(2)=0 and find the three factors of f(x) Found 2 solutions by CharlesG2, ewatrrr Answer by CharlesG2(4) (Show Source) You can put this solution on YOUR website!Graph f(x)=x^36x^211x6 Find the point at Tap for more steps Replace the variable with in the expression Simplify the result Tap for more steps Simplify each term Tap for more steps Raising to any positive power yields Raising to any positive power yields Multiply bySimple and best practice solution for F(x)=4x^26x8 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework




What Way We Can Solve This X 6x 11x 6 0 Quora




Overview Of Rational Zeros Of A Polynomial Online Math Tutor Youtube
Find stepbystep Engineering solutions and your answer to the following textbook question Determine the highest real root of f (x) = x^3− 6x^2 11x − 61 (a) Graphically (b) Using the NewtonRaphson method (three iterations, x_i = 35) (c) Using the secant method (three iterations, x_{i−1}= 25 and x_i= 35) (d) Using the modified secant method (three iterations, x_i = 35,To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW `x^36x^211x6=0` In each of the following, g(x) is a factor of polynomial f(x) or, not f(x) = x^3 6x^2 11x 6, g(x) = x 3 asked Apr in Polynomials by Daivi ( 261k points) factorization of polynomials




Factor Factor A Polynomial And Trinomial With Step By Step Math Problem Solver




Apply Division Algorithm To Find The Quotient Q X And Remainder R X Obtaimed On Dividing X3 6x2 11x 6 By X2 X 1 Ate I And 2 Maths Polynomials Meritnation Com
X^2 5x 6 = (z2)(x3) Let f(x) = x^3 6x^2 11x a f(2) = 6 a For x2 to be a factor f(2) = 0, so a=6 f(3) = 6 a For x3 to be a factor, f(3) = 0, so a = 6 a=6 To check this,divide x^3 6x^2 11x 6 by x2 to get x^2 4x 3,Zeroes of x3 −6x2 11x−6 By using rational theorem, the roots can be among the factors of 16 =6 Let us try x =1 ⇒ (1)3 −6(1)2 11(1)−6 =0 ∴ (x−1) is a factor of x3 −6x2 11x−6 Now, using synthetic division method So, the quotient =x2 −5x6Solution for f (x)=11 equation Simplifying f (x) = 11 Multiply f * x fx = 11 Solving fx = 11 Solving for variable 'f' Move all terms containing f to the left, all other terms to the right Divide each side by 'x' f = 11x 1 Simplifying f = 11x 1



Solved One Factor Of The Function F X X3 6x2 11x 6 Is X 3 Describe How To Find The X Intercepts And The Y Intercept Of The Graph Of F X Wit Course




Verify Rolle S Theorem For The Function F X X 6x 11x 6 On 1 3 Maths Continuity And Differentiability 1375 Meritnation Com
x^36x^211x6=color(red)((x1)(x2)(x3)) There are several ways to approach this One of the most reliable is to hope that the expression has rational roots and apply the Rational Root Theorem In this case, the Rational Root Theorem tells us that (if the expression has rational roots) those roots are integer factors of 6 (the constant term of the expression)




Divide The Polynomial X3 6x2 11x 6 By X 2 By Long Division Brainly In




Divide The Polynomial X3 6x2 11x 6 By X 3 And Check Whether X 3 Is A Factor Of The Polynomial Brainly In




If The Product Of Zeroes Of The Polynomial F X Ax 3 6x 2 11x 6 Is 4 Then A Youtube
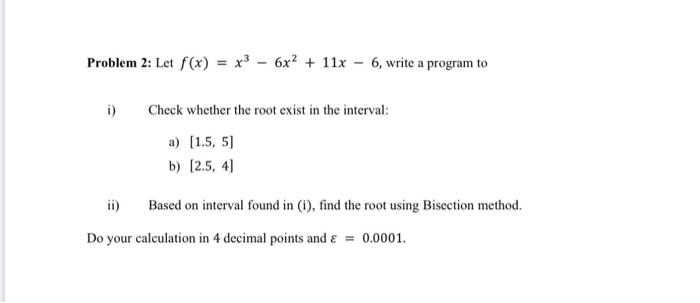



Problem 2 Let F X X3 6x2 11x 6 Write A Chegg Com




If F X X 2 4 X 3 6x 2 11x 6 X 1 X Then Set Of Points At Which The Function If Non Differentiable Is




Divide X 3 6x 2 11x 6 By X 2 8x 27




3 Determine The Highest Real Root Of F X X3 6x2 11x 6 1 A Graphically B Using The Brainly Com
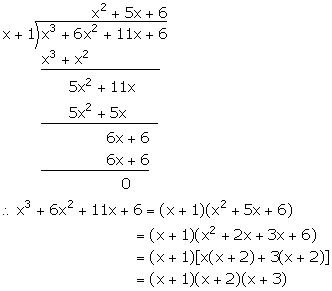



Chapter 8 Remainder And Factor Theorems R K Bansal Solutions For Class 9 Mathematics Maharashtra Topperlearning




Determine The Roots Of F X X 3 6x 2 11x 6 Study Com




Two Zeroes Of The Polynomial X3 6x2 11x 6 Are 2 And 3 Find The Third Zero Maths Polynomials Meritnation Com
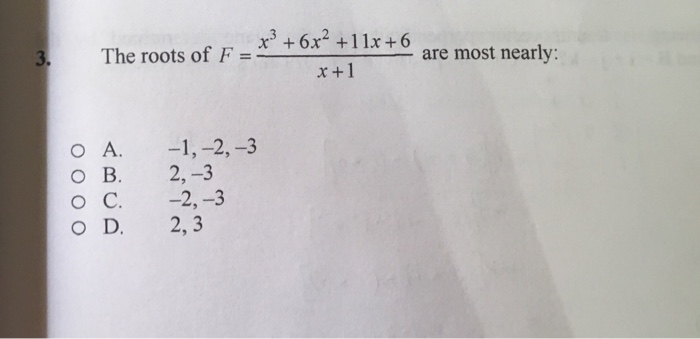



X3 6x2 11x 6 Are Most Nearly The Roots Of F 3 Chegg Com



P X X 3 6x 2 11x 6 G X X 2 5x 6 Sarthaks Econnect Largest Online Education Community




14 Simplify 1 8 V2 10 V2 912 Ii 3 V3 Liju 15 Using Factor Theorem Factorise The Polynomial X3 6x2 11x 6 1 On Line




2
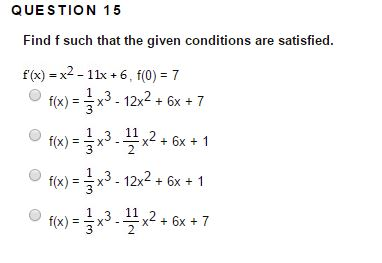



Find F Such That The Given Conditions Are Satisfied Chegg Com
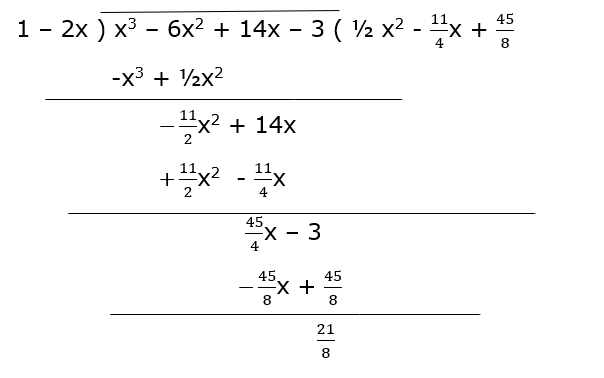



Ex 2 3 Q8 Find The Remainder When P X X 3 6x 2 14x 3 Is Divided By G X 1 2x
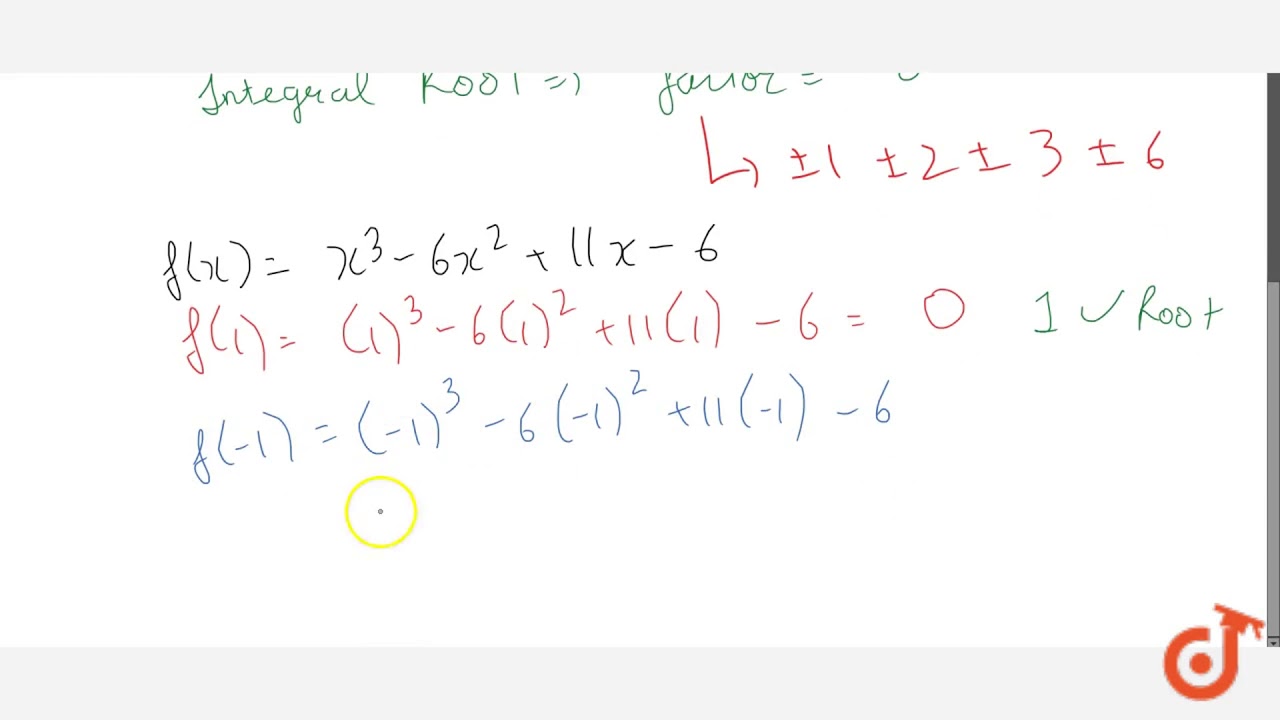



Find Integral Roots Of The Polynomial X 3 6x 2 11x 6 Youtube




Solve Each Of The Following Quadratic Equations 6x 2 11x 3 0 Youtube




X 3 6x 2 11x 6 0 Youtube
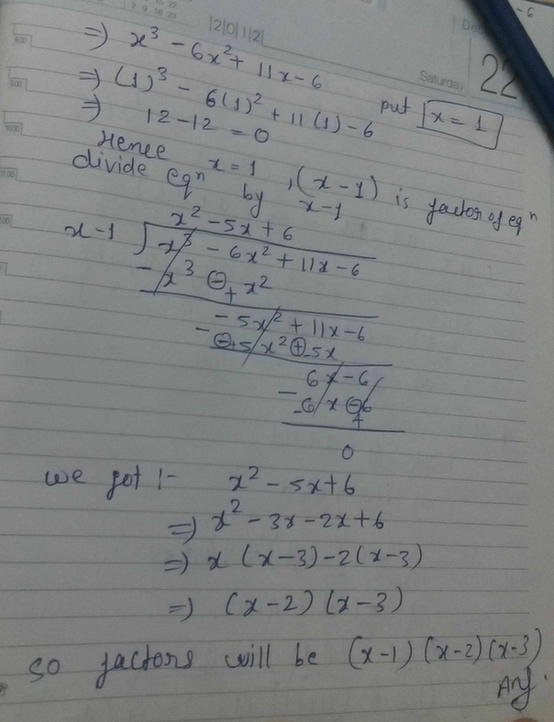



X 3 6x 2 11x 6 Scholr



If One Zero Of The Polynomial P X X3 6x2 11x 6 Is 3 Find The Other Two Zeros Brainly In
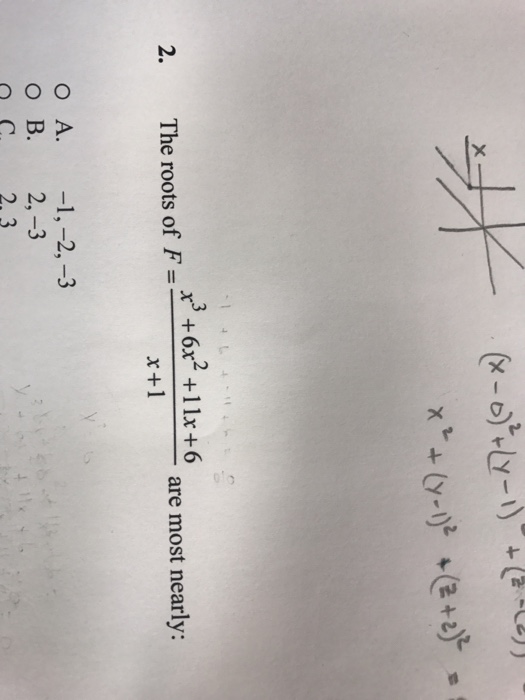



The Roots Of F X 3 6x 2 11x 6 X 1 Are Most Chegg Com
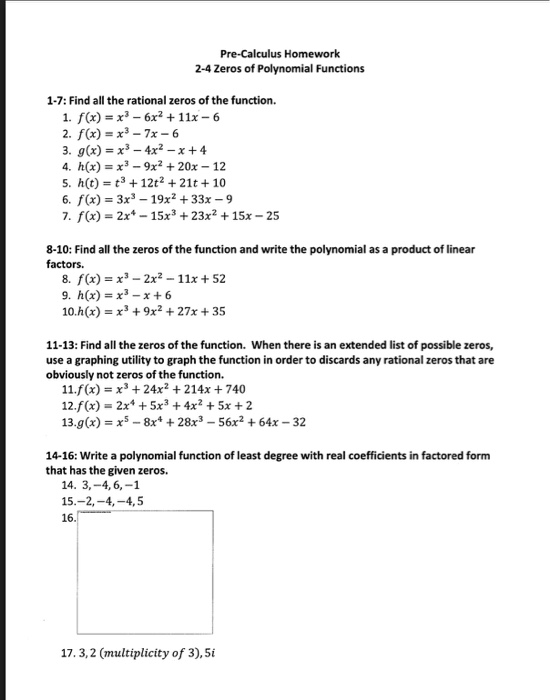



2 4 Zeros Of Polynomial Functions 1 7 Find All The Chegg Com




How Do You Use The Graph Of F X X 3 6x 2 11x 6 To Rewrite F X As A Product Of Linear Factors Socratic




Numerical Methods For The Root Finding Problem Pdf Free Download




Solve The Following Equations X 3 6x 2 11x 6 6 Youtube



Q Tbn And9gcq2vw Cnaruq0xbbzn8dooyjhdnac0qzirgfbptdhrktyo7saio Usqp Cau
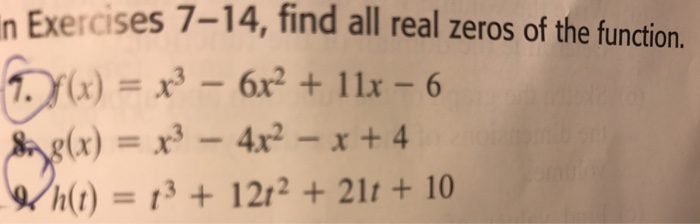



Find All Real Zeros Of The Function F X X 3 Chegg Com




Find The Integral Roots Of The Polynomial F X X 3 6x 2 11x 6



Mathscene Factorising Polynomials Lesson 1




Let F X X 2 3x 2 X 3 6x 2 11x 6 Sin X Pi 4 Number Of Points At Which The Function F X Is Non Differentiable In 0 2 Pi Is
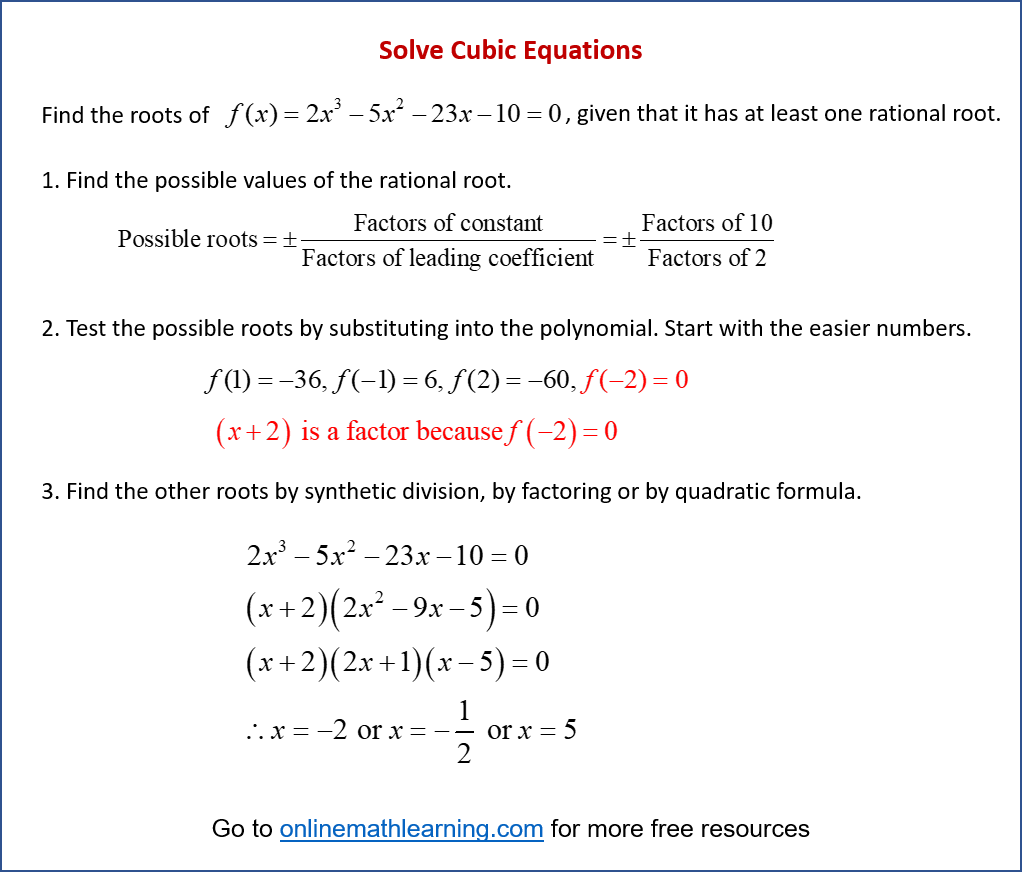



Solving Cubic Equations Solutions Examples Videos



What Is The Value Of X In The Equation Math X 3 6x 2 11x 6 0 Math Quora
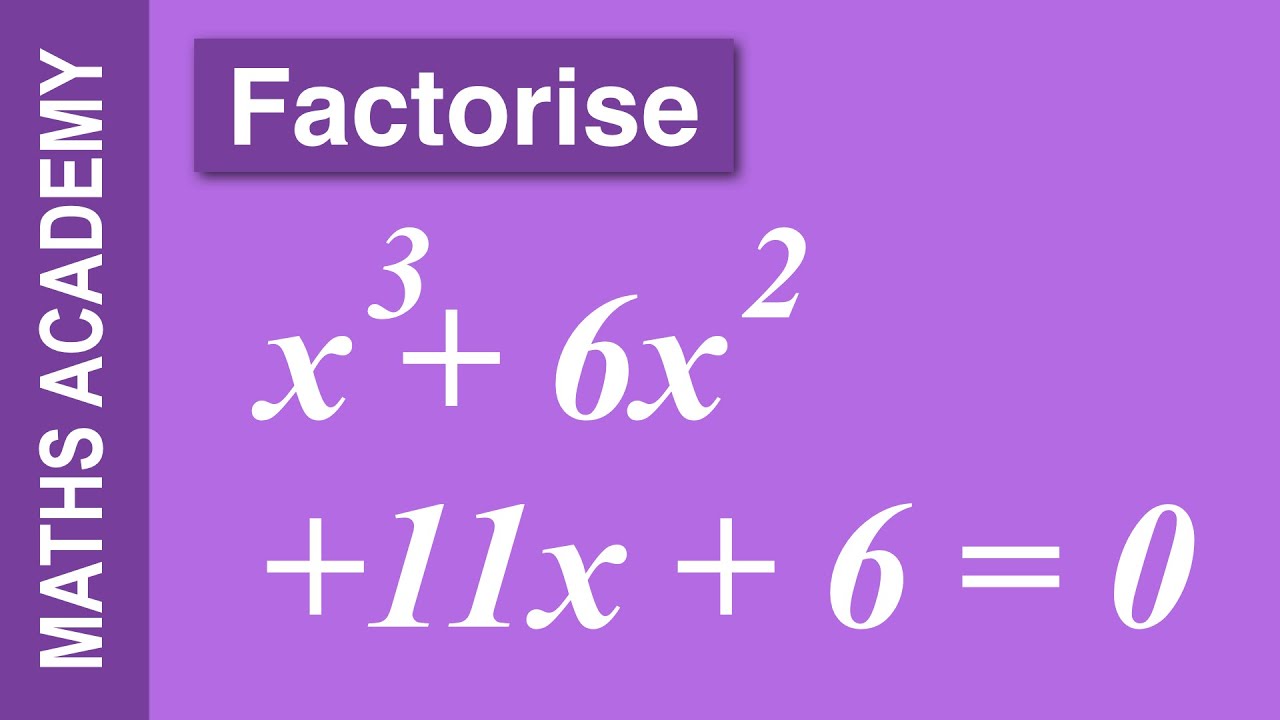



How To Factorise X 3 6x 2 11x 6 0 Youtube




If One Zero Of The Polynomial P X X 3 6x 2 11x 6 Is 3 Find The Other Two Zeros




Solve The Following Equations X 3 6x 2 11x 6 6
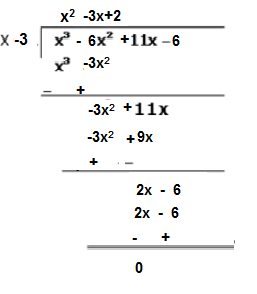



If One Zero Of The Polynomial P X X3 6x2 11x 6 Is 3 Find The Other Two Zeros Snapsolve




Apply Division Algorithm To Find The Quotient Q X And Remainder R X Obtaimed On Dividing X3 6x2 11x 6 By X2 X 1 Ate I And 2 Maths Polynomials Meritnation Com




F X X 3 6x 2 11x 6 G X X 2 Brainly In




If One Zero Of Thepolynomial P X X3 6x2 11x 6 Is 3 Find The Other Two Zeros Brainly In
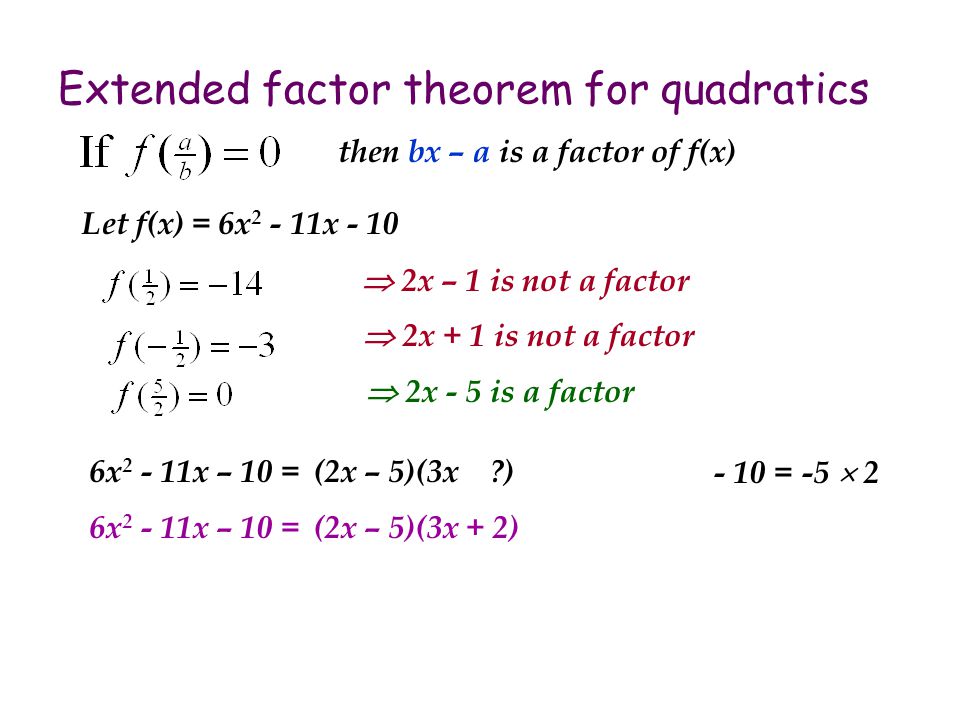



The Factor Theorem The Factor Theorem States That If F A 0 For A Polynomial Then X A Is A Factor Of The Polynomial F X Example F X X 2 X




Factorise X3 6x2 11x 6 Completely Using Factor Theorem Mathematics Shaalaa Com
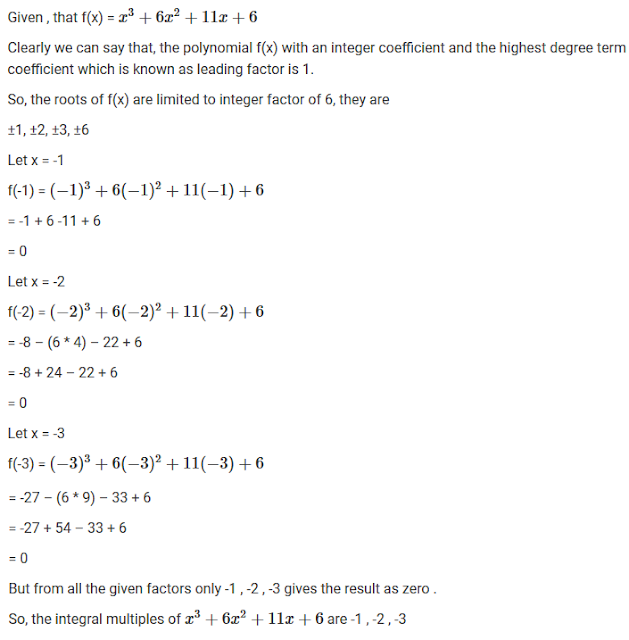



R D Sharma Solutions Class 9th Ch 6 Factorization Of Polynomials Exercise 6 2




बह पद X 3 6x 2 11x 6 क एक ग णनख ड ज ञ त क ज ए




Copyright C 09 Pearson Education Inc Chapter 4 Polynomial And Rational Functions 4 1 Polynomial Functions And Models 4 2 Graphing Polynomial Functions Ppt Download
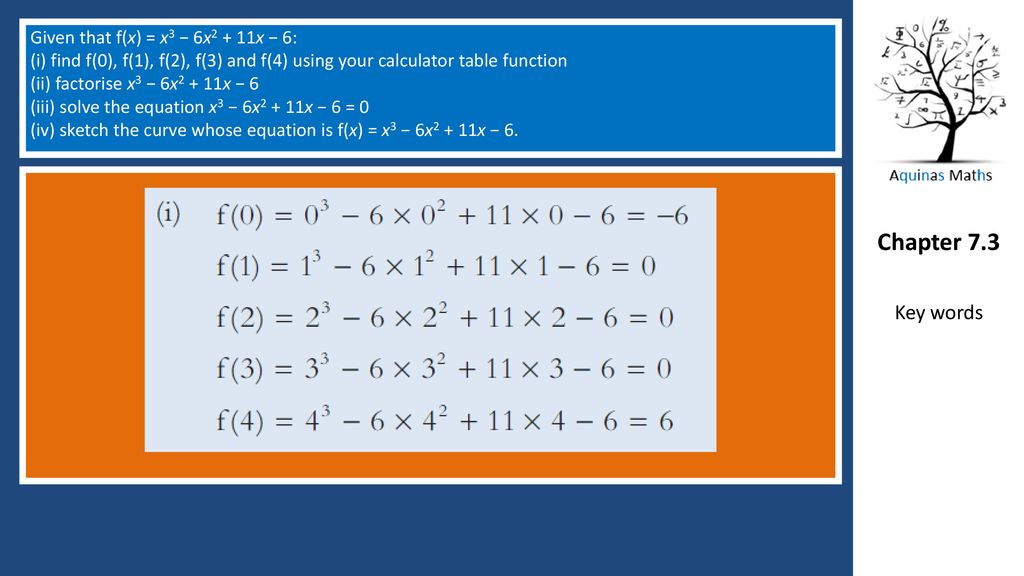



Polynomial Expressions Ppt Download




Factorise P X X3 6x2 11x 6 Maths Polynomials Meritnation Com




Determine The Root Of X 3 6x 2 11x 6 1 0 Chegg Com




Lim X 1 X 2 3x 2 X 3 6x 2 11x 6




Find The Root Of The Equation X 3 6x 2 11x 6 0



F X X 3 6x 2 11x 6 G X X 2 X 1 Sarthaks Econnect Largest Online Education Community



It Is Given That X 2 5x 6 And X 3 6x 2 11x A Have A Common Factor What Is The Possible Value Of A Quora




Find The Integral Roots Of The Polynomial F X X 3 6x 2 11x 6 Youtube



F X X 6x 11x 6 G X X X 1 Find The Quotient Sarthaks Econnect Largest Online Education Community
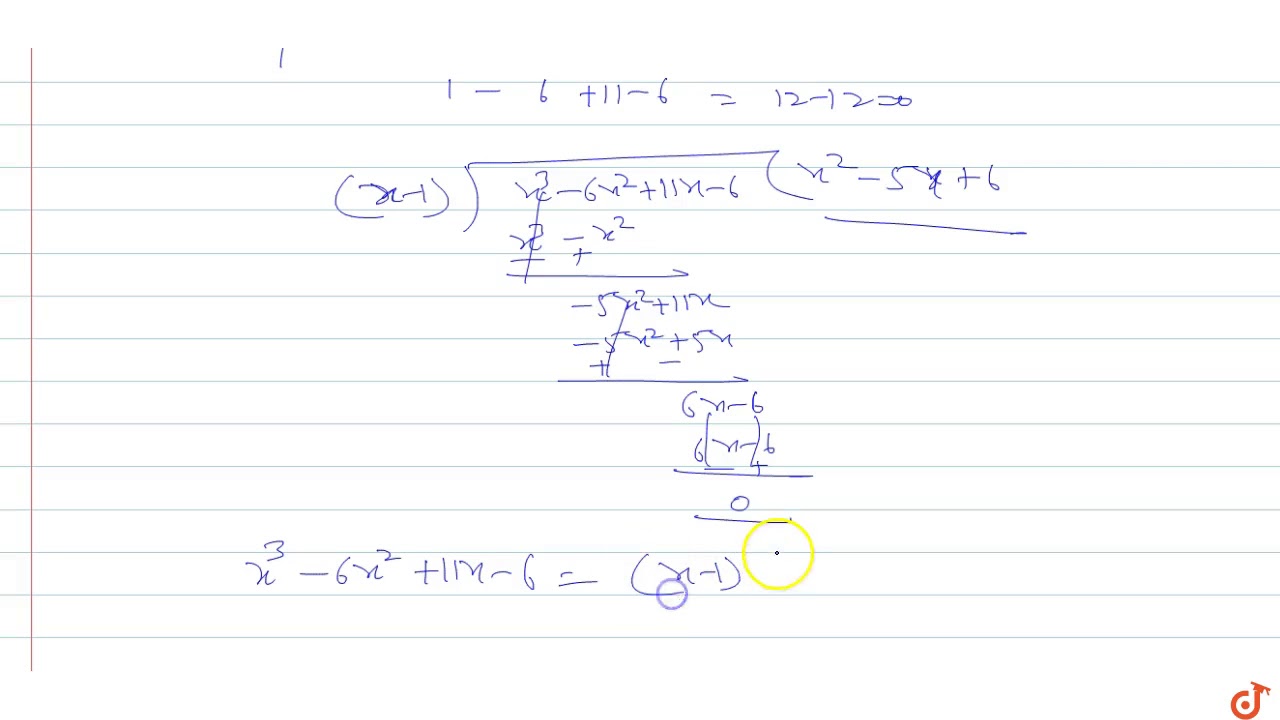



Factorize X 3 6x 2 11x 6 Youtube



Consider The Function F X X3 6x2 11x 6 If F X 0 For X 3 For What Other Values Of X Is The Brainly Com




Ex 3 2 Q1c F X X3 6x2 11x 6 G X X 2 Find The Quotient And The Remainder On




World S First One Variable Periodic Functions Utilizing Polynomials Such As X 3 6x 2 11x 6 Math Focus Series Book 7 Plutonium Archimedes Amazon Com




How To Factor Math X 3 6x 2 11x 6 0 Math Quora



It Is Given For The Function F X X 3 6x 2 Ax B Rolle S Theorem Holds In 1 3 With C 2 1 3 Find The Values Of A And B Sarthaks Econnect Largest Online Education Community




Factorise X 3 6x 2 11x 6 Using Factor Theorem And Long Division Method




Answered Applicatinns Of Differntiation Chapter Bartleby




Factorize X3 6x2 11x 6 Mathematics Topperlearning Com Iv3dg99oo



Mathscene Factorising Polynomials Lesson 1



Factorise X 3 6x 2 11x 6 Completely Using Factor Theorem Sarthaks Econnect Largest Online Education Community




If One Zero Of The Polynomial P X X3 6x2 11x 6 Is 3 Find The Other Two Zeros Brainly In




Divide F X X3 6x2 11x 6 By G X X2 X 1 Using Division Algorithm Youtube




Divide X 3 6x 2 11x 6 By X 2 5x 6 Brainly In




Divide X 3 6x 2 11x 6 By X 2 And Verify The Division Algorithm
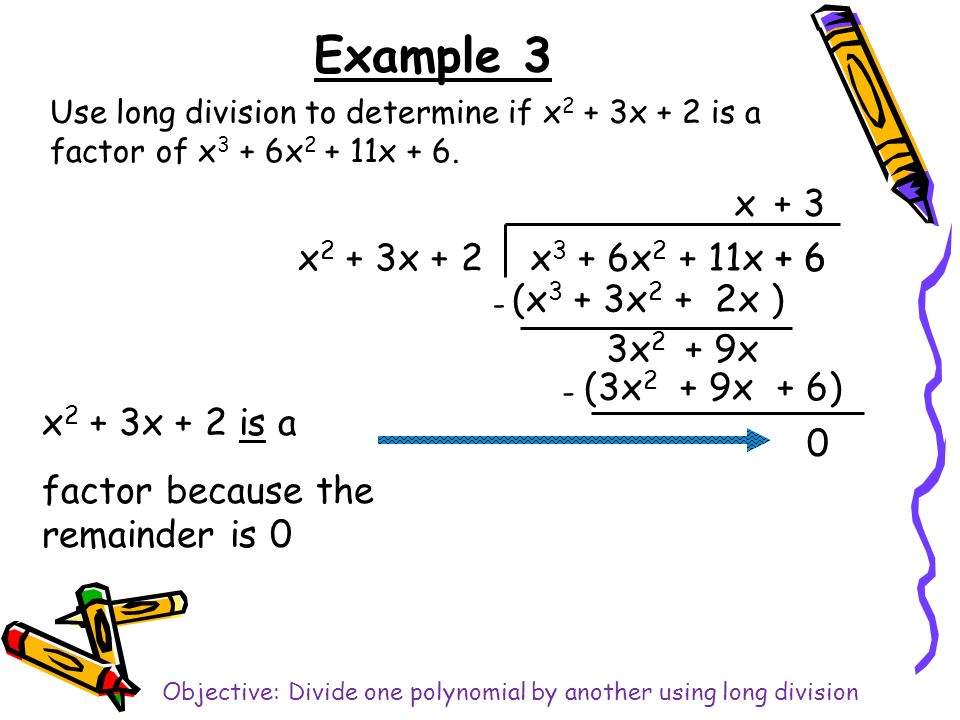



7 3 1 Products And Factors Of Polynomials Products And Factors Of Polynomials Objectives Multiply And Factor Polynomials Use The Factor Theorem Ppt Download
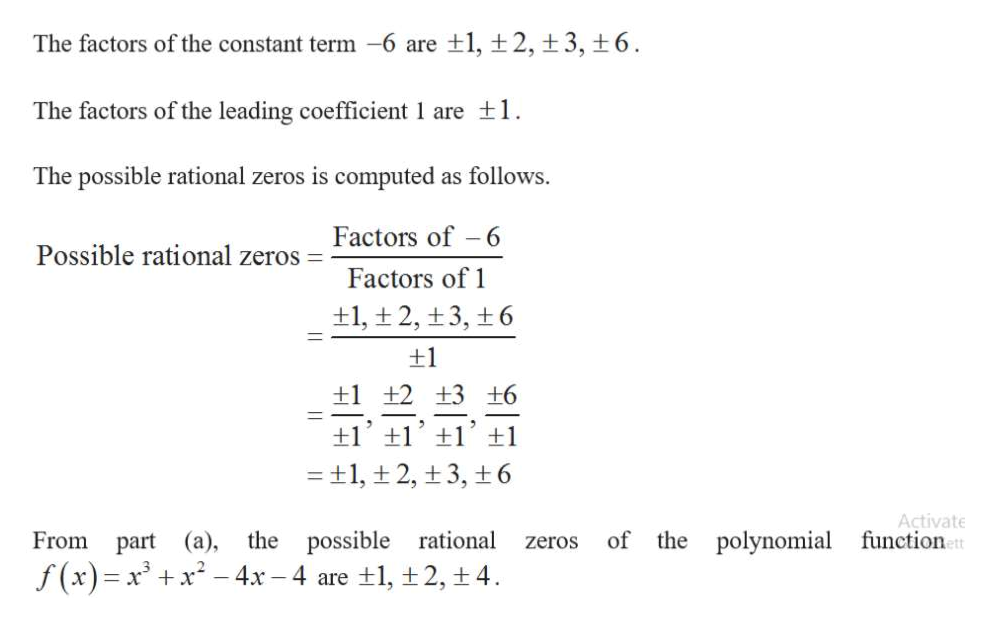



Answered Find All Rational Zeros Of The Function Bartleby




If F X 3 6x 2 11x 8 X 2 1 Find The Sum Of Possible Values Of F 14 Maths Relations And Functions Meritnation Com



How Do You Factor X 3 6x 2 11x 6 Socratic




Use The Remainder Theorem To Completely Factor P X X3 6x2 11x 6 Brainly Com




Divide X 3 6x 2 11x 6 By X 2 X 1




Solve This Find The Integral Roots Of The Polynomial Fx X3 6x2 11x 6 Maths Polynomials Meritnation Com



0 件のコメント:
コメントを投稿